Quantum Computing – What is it about?
General Idea
Before being observed Schrodinger’s cat does not need to decide which of two states of life (being dead of alive) it actually exists. It lives in a superposition of two states. And it is not a matter our or its ignorance. On some scales typical to single atoms, photons, and not only, under some conditions, nature simply does not have to choose.
The metaphor of the Schrodinger cat describes the concept of a quantum bit or qubit that is the unit of quantum information commonly used in quantum information theory and in quantum computing.
Fundamental features that distinguish quantum computing from classical computing are seen when we consider several qubits. For example, three of them unobserved can be in a superposition of 2^3=8 states. This is the number of all different states of three bits. The difference between three bits and three qubits is schematically shown in the figure below. While three bits at a given time must be in exactly one of 8 states, qubits do not need to decide. They can be in a superposition of all at the same time. Moreover, the amplitudes of different states in the superposition are given by non-binary complex numbers.
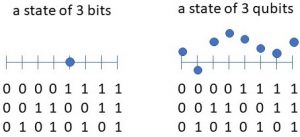
To describe a state of three bits three binary numbers are enough (left scheme). To describe a state of three qubits we need 2^3 complex numbers.
In consequence, while a state of three bits is described by just three binary numbers at each instance of time, describing a state of three qubits requires 2^3=8 complex numbers.
To follow processing of N bits we need to track N binary numbers, while for N qubits we need to keep track of 2^N complex numbers. It becomes heavy for the memory and the computational power of standard computers when N is still relatively low (say N=30). Yet nature easily handles the evolution of hundreds of qubits which corresponds to evolution of medium size molecules.
So, the main idea of quantum computers is to take a quantum system with N qubits and design an evolution that naturally processes them which is equivalent to manipulate 2^N amplitudes. The amplitudes may encode a real problem and its evolution may realize a high complexity computation that exceeds the abilities of classical computers. Yet a quantum computer may deal with the problem as easily as nature deals with molecules.
Current State of the Art and Challenges
Realizing a functional quantum computer requires many sides playing together:
- Hardware. Quantum computers are currently developed on several platforms including superconducting qubits, ions, photonics, etc.
- Algorithms. Quantum computing requires special algorithms that can act on all amplitudes in parallel. Algorithms of classical computers cannot be easily translated to quantum machines. We already know a few algorithms that offer a quantum advantage, but there is still a lot to do in this matter.
- Error correction. Quantum superposition is extremely sensitive on thermal noise, losses, and many kinds of errors that appear in real devices. The superposition can be gradually lost and the system reduced to a stochastic system. Moreover, we can not apply traditional error correction as we can not measure the state before the computation is finished, otherwise the superposition is lost. Alternative error correcting schemes are needed and developed.
- Applications. We know several fields which can potentially benefit from quantum computing. This may include predicting evolutions of quantum system like in quantum chemistry or material science based technology. Also, application of quantum computing on small and noisy devices for machine learning is intensely investigated. Currently it is hard to predict precisely the range and fields of applications. They depend on the development of the items listed above.
Our Contribution and Interests
In our Quantum Information and Communication Group we explore fundamental aspects of quantum computing and their potential applications. Specifically:
- We investigate abilities and limits of hardware based on quantum states of light.
- We participate in development of the so-called boson sampler for quantum chemistry at Keio University. We are interested in developing hardware as well as recognizing limits of application of this technology.
- We participate in development of photonic devices for machine learning.
- We are interested in applications of boson sampling for high complexity graph theory problems.
- We are improving optimization algorithms for machine learning with quantum photonic devices.
- We are interested in applications of quantum annealers for compressive sensing.
Find out more about our research in this field checking our Publications or Contact us directly.